Equation notes
These notes represent one approach to introduce solving equations. I would typically teach this to year 8 students, who have previously studied fractions, decimals, and negatives. Discussion of these problems and their strategies will often yield the following; trial and improvement, collecting terms, cancelling from both sides, and checking solutions by substitution. Things I emphasise are correct use of equals sign, equations as statements of equality, and solutions as values of variables that make statements true. I also think this approach sets up algebra as a construct for solving problems. The lesson numbers are only to give a sense of possible timings, this is not intended to be prescriptive in anyway.
Lesson 1
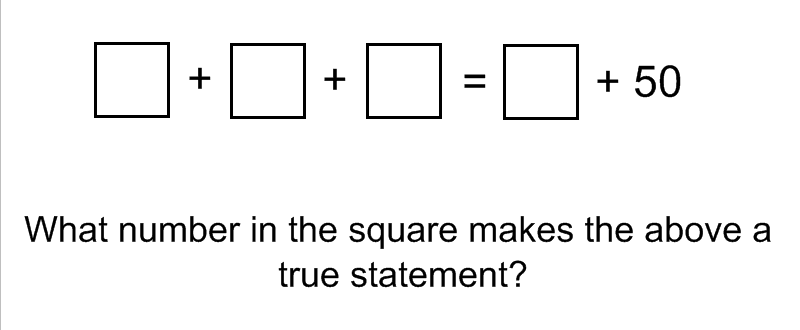
The problem below is posed with no explanation, this works well displayed with students working on whiteboards so they can change values easily. I have found many students will complete with different values in the boxes, I will then pose it as a challenge to complete using same number in each empty box.
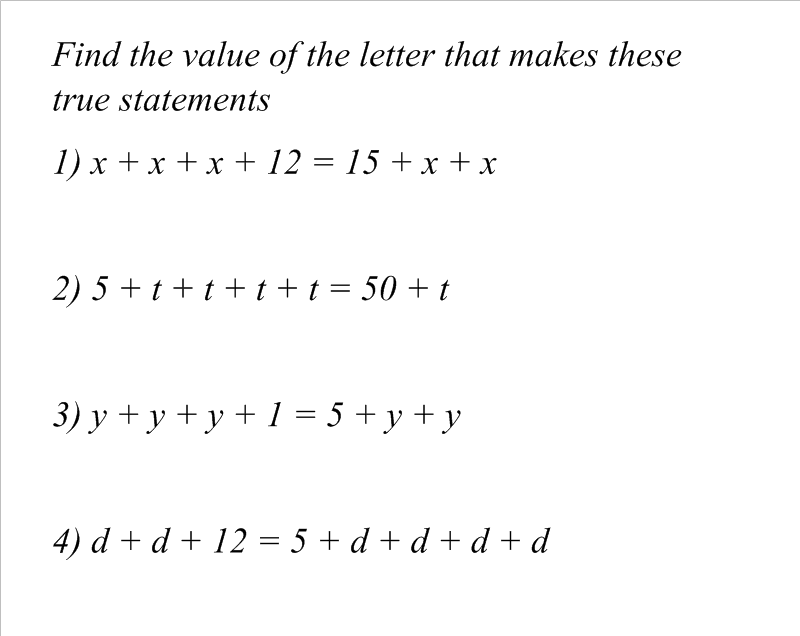
Once students have found the solution to the first one, they are given the one below. This time printed as students will require at different times.
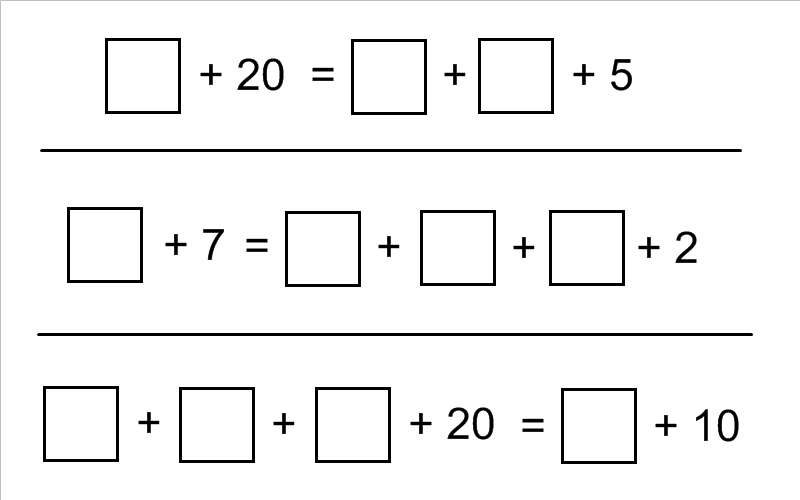
Students are encouraged to articulate their thoughts at this stage, prior to group discussion. Whilst facilitating this discussion I would be aiming to attention towards; the meaning of equals sign, the process of checking solutions, and the definition of a solution.
Lesson 2
Students work through the following tasks, interspersed with discussions and opportunities for students to write reflections. Again I emphasise are correct use of equals sign, equations as statements of equality, and solutions as values of variables that make statements true. During this lesson I circulate providing extension, this is often in the form of posing problems to introduce another concept (such as fractions, negative coefficients, indices) and support, often by drawing attention to method in previous questions. I will have resources for all lessons printed so students can move on at different paces.
Discussion following these needs to include why boxes are ambiguous (may represent different values), hence use of letter where same letter means same value within a question.
Collecting of terms made explicit at this stage to students.
Lesson 3
Mixed questions, often used as self assessment for students, also aids in making the previous links more explicit.
Practice at this stage will be a mixture of students generating questions, questions provided, and students explaining their thinking.
Lesson 4
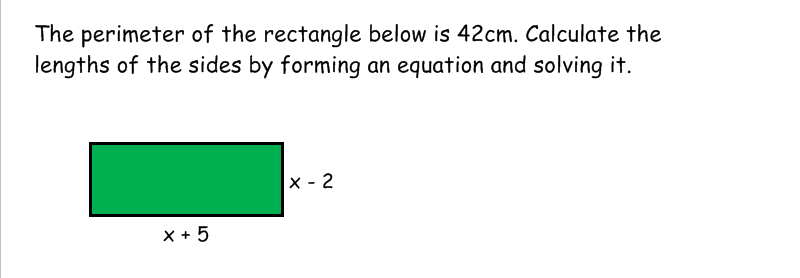
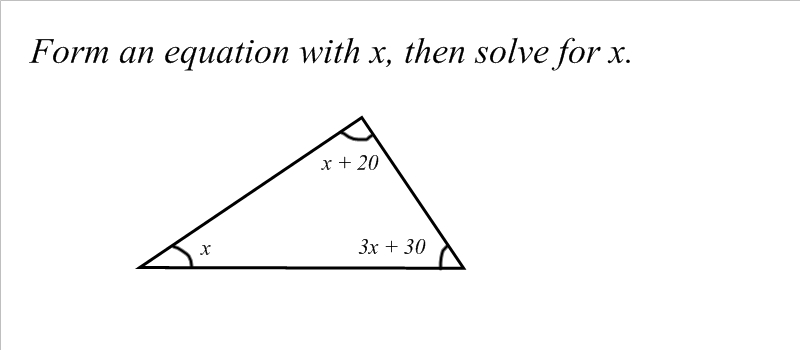
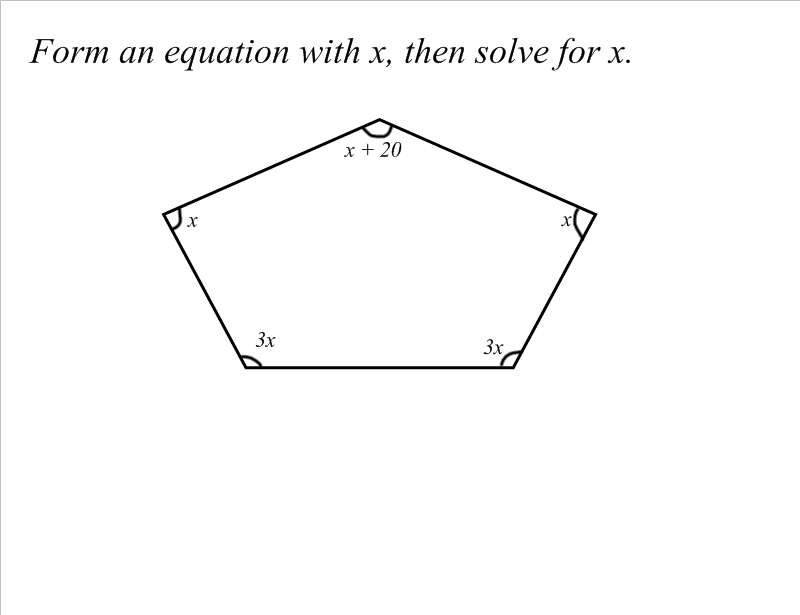
Tasks that encourage students to form equation. Although many students will be able to solve some of these without forming an equation, I ask students to do so.
Here is flipchart for these.



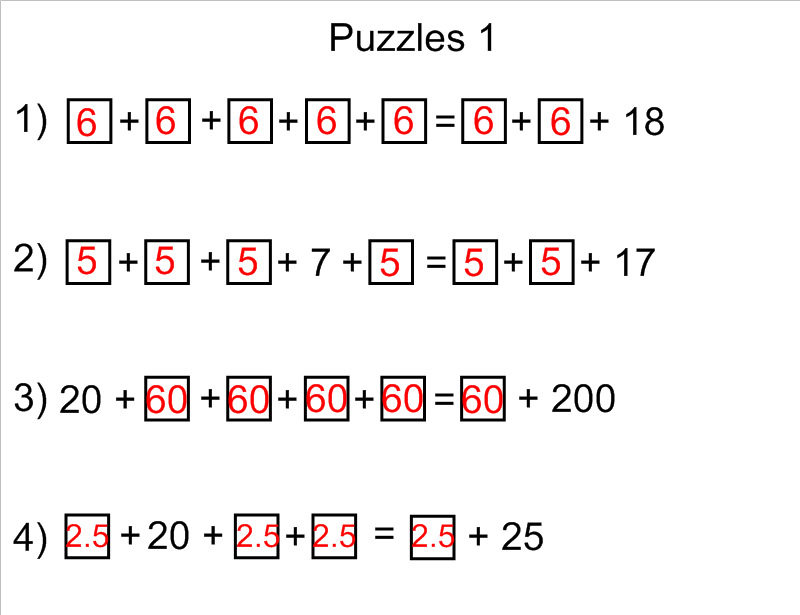
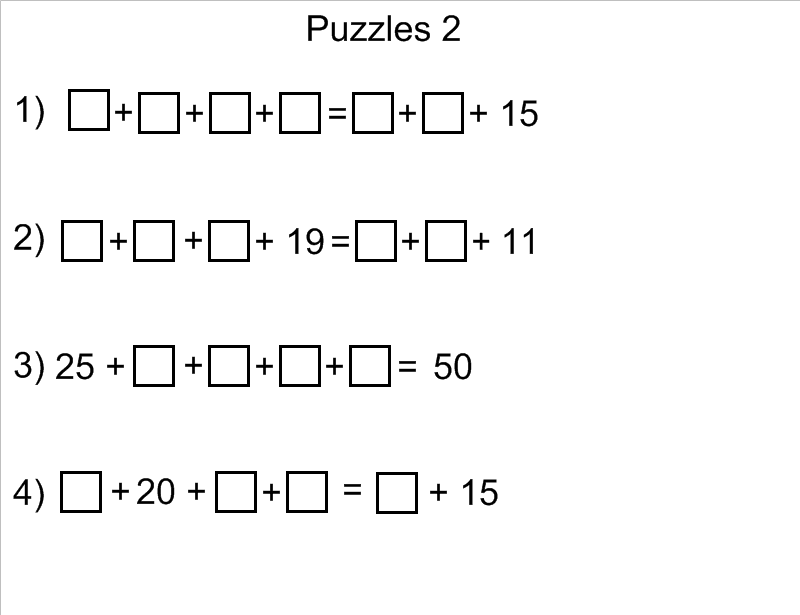


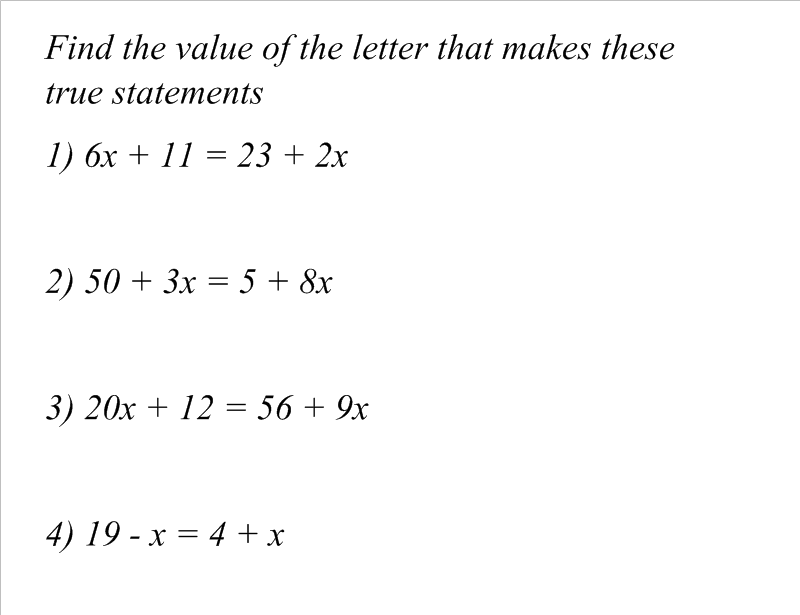
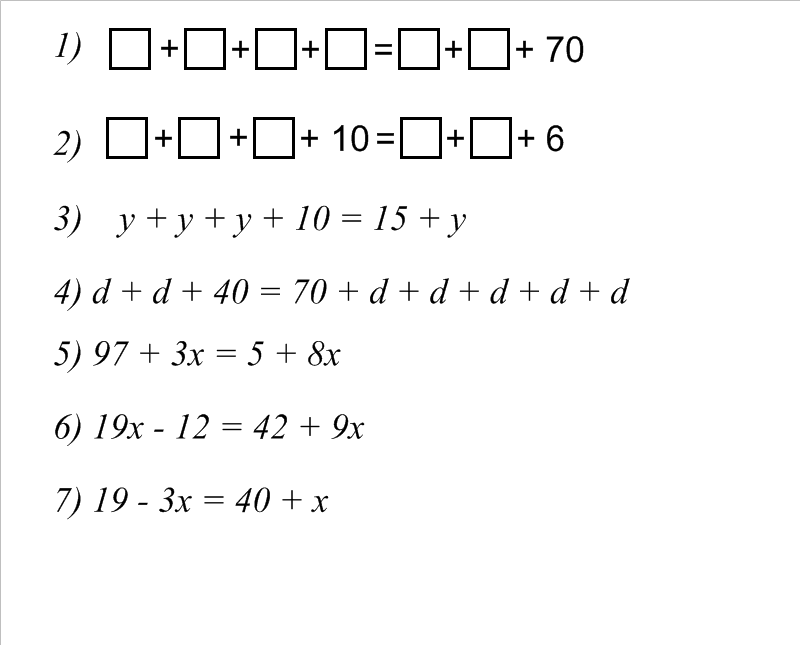




Hi Sam
I think the initial approach is a good idea, and hopefully will give students the opportunity to become aware of the equivalence of e.g. 3x and x+x+x… and also perhaps reveal an awareness of some of the x’s being ‘duplicated’ on both sides, which might later suggest a method for solving equations (subtracting x’s from both sides).
Perhaps some subtraction or multiplication between the boxes might be interesting here too as an opportunity for generalisation? [i.e. does the method you are using to fill in the boxes still hold for these other operations?] But perhaps this would over-complicate, or take the focus away from the awarenesses you wish to ‘force’.
I can see you have tried to introduce a ‘reason’ why we (students) might want to solve such equations in lesson 4, but they are still sort of ‘arbitrary’ questions. I wonder if there is some kind of investigation (perhaps starting with one of the geometric examples and extending/generalising it) that would achieve this whilst at the same time require the use of some other mathematical skills such as conjecturing. generalising, and so on?
D
Thanks Danny, I wasn’t aware that I was presenting a reason for solving equations in lesson 4, but see now that students possible think I am. Rather I wanted to mixed with prior topics studies (area and angles) and reinforce this idea of equations as statements, so going from the geometric statement “interior angles in a pentagon sum to 540 degrees” to an algebraic statement “x + x + 30 + 3x + x + 3x = 540”. If you have any ideas for activities / investigations I would love to hear.
Hope your well,
Sam
I really like the approach to solving equations through puzzlement and missing numbers. Pedagogically is a lovely mix of accessibility and challenge with clear developments; if I were writing a SoW I would certainly use the kind of ideas you offer here. I also like the use of algebra appearing in other concepts, e.g. angle. I have ‘Yes and’ to suggest which is something I always seek to weave into learners’ mathematics experiences; this is to provide them with opportunities to make up their own questions for others to solve. My experience shows they sometimes come up with even harder equations. E.g. 🔲+🔲+🔲x🔲-🔲/2 = 10. However this is not the main reason; it is so they gain greater ownership of the work they do and they (the cross-KS2 class I teach once a week) love trying to ‘test’ me out!
Thanks Mike, students creating their own questions is a lovely idea, I hadn’t given much thought to the idea of ownership. I often use this with some restraints (eg form an equation with solution greater than 500 or a negative, solution), at some stage this will usually involve me sharing my “teacher trick” of starting with the solution and building up. For me this is about the idea of doing and undoing that leads to more fluency of manipulations.
Cheers, Sam
The business of ‘Doing and undoing’ permeates mathematics per se and, as such, the T&L of mathematics ought similarly be ‘drenched’ in “D&U”. Some work I did with a KS2 class involved the following processes:
1. I asked everyone to draw a rectangle on cm squared paper whose dimensions were 2. As part of this I asked them to explain what ‘dimensions’ meant so everyone used w and h for width and height of their rectangle.
2. Next I asked them to calculate the area and perimeter of their rectangles, referring these measures as A and P. This brought about misconceptions about how to calculate the perimeter of a rectangle when different children explained their methods. Having sorted out which of their three methods was correct we moved on.
3. Now each child had a rectangle with four pieces of information: w, h, A and P.
4. I asked them to make a sketch of their own rectangle on plain (non-squared) sugar paper showing just two of the four pieces of information, i.e. h and A or w and P or A and P, together with their name. So for example Lily drew her rectangle and wrote w = 6 and A = 30.
5. Everyone stuck their piece of sugar paper around the classroom walls and everyone then had to work out the two missing pieces of information from a selection of the data around the room.
Thus each child engaged with doing and undoing. A further key pedagogic issue was how their collective pieces of sugar paper formed a ‘worksheet’ which they collectively had ownership of. This was a far more powerful aspect of their learning by contrast to any worksheet I might have given them to do.